Research Overview
Our current research aims to theoretically model and understand the nonequilibrium phenomena in complex dynamical systems. We focus on the structural evolution and assembly in solid and soft materials and active matter, formation and dynamics of complex patterns and microstructures involving effects of elasticity and plasticity and topological defects, as well as nonlinear dynamics in biomedical systems. Example systems include, but not limited to:
- Strained solid films, such as epitaxial heterostructures with spatial and compositional modulations and/or topological defects;
- Microstructural dynamics, topological defects (dislocations and grain boundaries), ordering, and mechanical properties of novel 2D materials;
- Pattern-forming nonpotential systems far from equilibrium, particularly nonrelaxational dynamics in active patterns/crystals of self-propelled and/or self-spinning particles, and in fluid convection patterns with the emergence of spiral defect chaos.
Most of our efforts are based on the development of efficient multiple-scale methods for modeling the above nonequilibrium systems. It involves approaches and analyses at different levels/scales of description that incorporate continuum density-field theory and microscopic details of crystalline/periodic structures, defect configurations, or nanophases. Examples include the phase field crystal (PFC) method, “slow”-scale amplitude equation formalism, mesoscopic-level coarse-grained modeling, and hydrodynamic coupling.
Structural dynamics, topological defects, and ordering in 2D materials
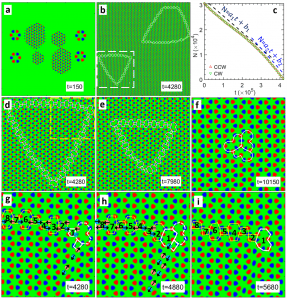
Left: Grain coalescence and collective dynamics of inversion domains (60-degree grain boundaries) in the PFC modeling of binary 2D hexagonal material such as hexagonal boron nitride (hBN).
(see Phys. Rev. Lett. 118, 255501 (2017))
Below: Chiral vs achiral 2D phases and superlattices.
(see Phys. Rev. Lett. 116, 205502 (2016))
Nanostructure dynamics and defects in strained films
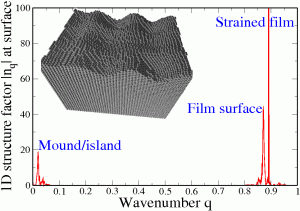Strained film heteroepitaxy, including a wide variety of epitaxial growth techniques such as MBE, CVD, VPE, LPE, etc., has become one of the most important methods in the manufacturing and processing of material nanostructures (including strained islands or quantum dots, nanowires, etc.). Our focus is put on understanding the key effects on the control and organization of such nanostructures in both single-component and alloy films. This involves the stress-induced effects and microstructures either on the film surface or across the interface, the coupling between morphological profiles and compositional inhomogeneity, interfacial diffusion vs surface mass transport, and the dependence on various growth parameters (e.g., temperature and growth rate).
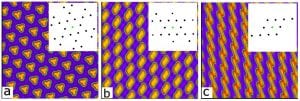
Defect dynamics and structural vs compositional evolution in strained solid films, which are among the main factors that strongly affect material properties, functionality, and potential device applications. Our research is focused on the coupling and interaction among topological defects (particularly grain boundaries and dislocations), phase separation in alloy films (with an example shown on right), and film surfaces or interfaces. We also emphasize the interplay between slowly varying profiles of interface, defects, or composition and the underlying small-scale crystalline structure, yielding important effects of lattice pinning with significant impacts on structural dynamics.
Complex pattern formation and defect dynamics
The spontaneous formation of complex modulated structures or spatiotemporal patterns is ubiquitous in both natural and synthetic material systems. Among the least understood are spatially extended systems driven far from equilibrium, for which the governing dynamics is nonrelaxational. One of our interests is in addressing the fundamental mechanisms underlying the complex phenomena of ordered vs chaotic pattern evolution in such nonequilibrium, nonpotential systems, such as the prototypical system of Rayleigh-Bénard convection. Stability, motion, and interaction of individual defects are found to play a key role in the transition to the state of spiral defect chaos, and also in the possible persistent dynamics of the pattern.
